Author: mrdardy

A Brief Post – Not About my Classroom

I am in my twelfth year at my current school and my family has lived on campus the whole time. We spent our first six years here living in a boys’ dormitory with over 80 teenage boys from all around the world. I enjoyed my time in the dorm but after six years we graduated to a campus home two blocks away from my classroom building. A few weeks ago my son decided, around 11 PM one night, that he wanted his head shaved the next day. He reached out to his friend text group to find out who was free during the last period of the day to help him with something. I, of course, did not find out about this until the next day when he casually mentioned that a friend would use my set of clippers to cut his hair during his free period at the end of the day. That friend happens to be one of my students, so I heard about this form him as well. During the course of the day word spread and a number of students who are free that period planned on coming over to watch and some livestreamed the event on Instagram. My son told me that as he was walking over to our house for this he was talking with some friends on the way. One of them made a remark to the effect of ‘this is why our school is so great.’ Now, of course, I could grumble and say that our school is great because of what goes on in the classroom, the stage, the athletic fields, the dorms, etc. I believe that there are a number of reasons why our school is a special place, but I realize that adventures like this one add a real spice to the day. The kind of communal, shared experiences, especially silly ones like this, help make being in a community more memorable and I have to realize that watching a friend shave another friends hair on a sunny early winter afternoon might be a more memorable experience than learning the Law of Cosines or something along those lines.

When my wife and I made the decision to move to a boarding school community I think that something along these lines was in my mind. The idea that my children get to be immersed in a community, they get to call their campus home as well as it being their school seems to be pretty powerful. I wonder whether they will become as sentimental about it as I am. Time will tell.

PS – It ended up being a pretty good haircut.

What can I do when I don’t know (remember) what to do?

My Calc BC classes had a test yesterday. We are deep in the midst of thinking about infinite series and I threw a question on the test that I thought would be a respite in the middle of some heavy lifting. I asked for my students to write a repeating decimal as a fraction in reduced terms. My two classes had slightly different numbers, I’ll concentrate my conversation here on this repeating decimal: 0.217217217217…

Many of my students remembered an approach where we called this number x and then created another number with the same repeating block. In this case, it would be 1000x as 217.217217217217…

A simple subtraction yields 999x = 217 and we have our desired fraction of x = 217 / 999

At least one student rewrote this as 0.217 + 0.000217 + 0.000000217 + … This student recognized this as an infinite geometric series whose first term is 217/1000 and whose common ratio is 1/1000. Remembering a nice formula gives us the same answer.

Some students fumbled on the problem not remembering either of these strategies. What I want to concentrate on here is the work of three students who all presented their reasoning essentially in the form of short paragraphs explaining how they zeroed in on this fraction. I won’t quote them directly and I will probably mix up their reasoning a bit after a long Friday.

One student presented the fraction 5/23 = 0.2173913 as a starting point. He admitted that this was after fumbling around with a couple of fractions all of which were ratios of primes. He reasoned that having no common factors would likely create ‘ugly’ decimals of some form. From there he mixed and matched some interesting reasoning. He showed that 2/9 = 0.222222… so he reasoned that 9s in denominators might be a nice thing. He showed something like 57/99 = 0.57575757… and this gave him confidence in targeting 217/999 for this problem. A different student started by pointing out that 1/5 < 0.217217217… < 1/4. This gave her an idea of where to start trying fractions that might zero in on the desired target. A third student presented some of these same ideas and concluded, somewhat apologetically, that he combined past knowledge and logic to arrive at his correct conclusion.

There is an awful lot to unpack in that particular explanation and it reminds me that one of my missions is to consistently champion such an approach to math. I displayed one of the papers on my document camera and made sure to publicly commend all three of these students. I pointed out that the ability to create this logic, to tie together past skills and ideas is FAR more meaningful than remembering some technique or formula. This is especially impressive in the time pressures involved in taking an in class test.

I had shared an old blog post in class recently when a student made a suggestion involving integration. The suggestion he made reminded me of a post I wrote a couple of years ago (you can find it here https://mrdardy.mtbos.org/2020/01/05/more-bragging/ ) and this prompted my students to notice that I am not blogging as much as I used to. They jokingly suggested that they were not worthy of blog posts. I need to make sure I use this space more frequently than I have been. I hope this is a kick start to more writing in 2022.

Oh yeah – a fun note about that post I linked above. I showed it to my students and one of them took a screen shot and forwarded to screen shot to the student I wrote about back in 2020. He replied while we were still in class together that he remembered that conversation from when he was in Calc BC!!!

Well, It’s been a while

I am out of practice at writing here, let’s see how this goes.

My last post was 14 months ago for all sorts of reasons, most of which have to do with the fallout of the pandemic. The spring of 2020 was sheer chaos as we were all trying to figure out how to teach virtually. The 2020 – 2021 academic year saw a multitude of rules restricting how we could interact with each other when we were in person (which, for our school, was the great majority of the year) and a couple of retreats into virtual schooling. I had students in front of me and students on my computer screen all year long (at least when we could have people in the same room!) and I know I was not alone in learning how to deal with that. I had all my students seated in rows and columns for the first time in 15 years and I had to try to relearn how to look out at a class like that. We could not sit in groups and share ideas with our neighbors at our elbows. I could not roam around the room and look over people’s shoulders and quietly share ideas and questions. None of this is news to anyone who is reading this. What I am trying to figure out is what tools/habits I’ve developed in the past fifteen months are worth carrying forward into the 2021 – 2022 academic year and beyond. There is a cliche about not wasting a crisis and I had some pretty meaningful conversations with my students about the ‘new normal’ this year. I laid out some practices that I had adopted, practices that were not part of my repertoire before COVID days. I asked them what was worth keeping and what they would be happy to never deal with again. The three features of life this year that got the biggest endorsements were (1) Scanning and submitting written work so that they could access their work and my comments at a later time. (2) My inclusion of DeltaMath into our life. (3) My use of BitPaper for classroom notes.

I want to think out loud about each of these three features.

1 – Obviously, any paper submitted and returned with notes/corrections/remarks is retrievable, even in COVID times. However, my students were really honest about their lack of organizational skills. Most papers that ever got returned got shoved into backpacks, ended up at the bottom of a locker, on the floor of their car or their bedroom, and were not accessible when it came time to study or reflect. I did not enjoy writing on their work through the google classroom and within the Kami environment. But if even a small portion of my students actually went back to the Google classroom page later to reflect, then it might (might!) be worth that time and effort.

2 – I was way too late to the DeltaMath game. I think Zach’s work there is tremendous and I got so much positive feedback from kids about the guided practice and videos available to them there (I paid for a plus membership so my students have limitless (I think it is essentially limitless) access to carefully presented videos to help them work out mechanical issues and to be reminded of why math life was unfolding the way that it was.

3 – I don’t know how many people know this tool. Here is an example of one of my BitPaper note pages https://bitpaper.io/go/Bell%206%20Calc%20Hon%20Week%20of%20May%2010/HJpuKAieh

I set up a new page for each section each week so that the pages would not get TOO cluttered. I did not write on a whiteboard at the front of the room at all this year. I did all of my writing on BitPaper and students had access to these pages at any time. There are some tweaks I wish they would make. I wish there was a scroll bar to move up and down the page. I wish multiple kids could view at the same time without interrupting each other. The second wish might be a lack of understanding on my part. But that is not what I want to write about. I want to talk about what I see as a huge advantage. My students can listen in on my conversation and what their classmates are saying. They can jot down quick notes or reminders to themselves, but they do not have to feel any pressure at all about transcribing while listening. They can look at class notes later and listen and think more efficiently in real time. I had a discussion about this tool and about the fact that I intend to use it again next year (or a different tool that might be better if someone can steer me to one!) and the response I got was that note taking is a vital skill and they need to work on this. I tried not to react negatively but inside I was sure feeling some serious skepticism about this claim. It feels obvious to me that listening and thinking and talking are more important than being a stenographer. If I am wrong here, if I am missing something important, I hope to hear it either in the comments or over on twitter where I am @mrdardy

There were SO many new tricks/tools that I tried this year but these three were the ones that resonated with my students. I would love to hear some reaction to these ideas and also hear about successful new tools/ways of thinking that infected your classroom during the pandemic.

Looks Like We Made It

Yesterday was the last day of classes for the 2019 – 2020 academic year at my school. I have an awful lot to unpack in the next couple of weeks about how the spring term unfolded. What I want to share tonight is how my last week went.

I teach five classes, three different preps. I teach one section of AP Calculus BC, two sections of Calculus Honors (our non-AP course in Differential Calculus), and two sections of Precalculus Honors. For my Calc Honors and Precalc Honors sections I set up a calendar for students to sign up for twenty minute ‘exit interviews’ with me. Last week on our google classroom page I posted a set of ten problems – a sort of mini final exam. I told my students that when they met with me I would randomly generate two numbers and they would explain those problems to me. It went pretty well. It was great having one on one time with each student and they mostly did a nice job of explaining problems that ranged throughout our year together. But what was fun was our brief conversations after the math. I asked each student to imagine that time travel was possible. I then said ‘What if I had taped this conversation over the last ten minutes and went back to August you to let her see what May you is up to?’ The reactions were pretty great. Almost every student said some for of ‘I’d be pretty amazed by what I can do’ or ‘I wouldn’t believe that I could make sense of those problems’ That, of course, was my point. I wanted each of them to have a brief, reflective moment where they thought about all that they had accomplished in the past nine months.

It has been a hard couple of months but I ended my day on Thursday all zoomed out (46 meetings in four days plus a couple of AP Calc group meetings) but I felt really good about this decision on how to end the year and about my silly time travel prompt.

Time to rest for a few days now…

Observations

Yesterday our school announced that we are to be physically closed until Monday, April 27. At that time we will re-evaluate our situation. Graduation is scheduled for May 24. This is hard news to digest. I understand that many many teachers and parents and students are digesting similar news right now.

At breakfast this morning my ten year old daughter said ‘I don’t like virtual school’. She then made a couple of remarks that I should remember exactly, but I don’t. I’ll paraphrase her – ‘I think that there should be some separation in our life. Home is for relaxing, snuggling with my kitties, and fun. School is where I focus and work on learning. It’s hard to do that in my room.’ What she did not touch on, but she definitely mentioned last week when all of this started for us is that school is where her friends are. FaceTime chats are fun, text chains can be as well. None of it replaces being around people.

I have made a commitment at this point (only four class days in so far) to hold zoom meetings for all of my 50 minute classes. For our 90 minute meetings I am setting up 10 minute one on one sessions. I don’t know how sustainable this all is, but the reassurance of seeing and hearing each other feels really valuable. Early survey results indicate my students feel the same way.

A Brave New World

This won’t be long, I don’t have enough emotional distance from today to make too much sense of what just transpired. I do feel it is important to at least register what this day felt like.

We were supposed to return to school from our spring break on Monday, March 16. Well, it did not work out that way. As many (MANY!) people are, we are dealing with uncertainty about when we will be a school community in a physical place together again. We took two days for intensive, in house PD aimed at easing the concerns of the adults on campus and aimed at beginning to develop some sense of comfort with our options. I chose to use Zoom as my vehicle for meeting with my students and we use a Google classroom environment to push and organize information.

We are on a rotating schedule and only three of my five classes were scheduled for today. We all met and of my 33 students that were supposed to be ‘with’ me, I saw all but three. Pretty darned good, especially considering that some of them are now scattered around the globe. One student logged in while waiting to go through customs at JFK and one student joined us from Korea at 3 AM local time there. Pretty amazing commitment from these young scholars. It was clear that they appreciated seeing and talking to each other again and it was great to see and hear them again. The interface is certainly not perfect. When there are over a dozen people, hearing anyone is tough. We need to get better at listening out for each other and paying careful attention to each other. I was physically aware of how draining it is to conduct a conversation where my eyes are scanning the thumbnails of 14 students at once, looking to see if they are engaged, if they need something from me. Over the course of 32 plus years of teaching, I think I have gotten good at recognizing those non-verbal cues from my students. It is much harder in this environment to find those cues, I will have to work hard (and fast) at this skill.

One of my colleagues had the idea of buying a graphics tablet to attach to his laptop. I am eager to hear how that goes. Writing with my touchpad is a sloppy mess. Any advice from experienced folks is more than welcome on this front!

I created some power point slides, ran a solo Zoom session and recorded myself narrating the slides. Not perfect, but the kids seemed to appreciate it. I did get a request for writing out my solutions rather than just talking through them. I may do some late night sessions in my classroom filming myself at the board. If so, what should be my vehicle for this?

My main takeaway from today as a dad of a 10th grader and a 5th grader as well as a teacher of 9th – 12th graders is that having some semblance of a normal routine was comforting. I think it is important for us to see and hear each other to maintain a sense that we are a community. I will be pretty insistent (and consistent) in asking for some community time when our class is scheduled to meet. We have a 90 minute class block immediately preceding a lunch hour. I can nab those times to schedule 10 minute one on one Zoom sessions to really check in on individual understandings. I think that any semblance of timed paper and pen assessments needs to be pushed aside, but I know that my school will have grade books open and they want some evidence of student work and some idea of progress. I have a lot of thinking to do in the next few days about this.

Certainly more questions than answers after today, but it felt great to see and hear my students again. Looking forward to the day when it is in person again!

As always, drop me a line here in the comments or over on the twitters where I am @mrdardy

More Bragging

Right before the Christmas break my Calc BC class was up tot heir elbows in new integration techniques. One of the old skills that comes into play often in this unit is the idea of long division as a way to rewrite rational expressions. All of my students have known how to do this but without having exercised that skill in some time, there is a bit of grumbling about it. I presented the following problem (or one very similar to it)

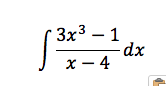
My intent was that my students recognize that they want to ‘reduce’ the fraction so that the numerator is a lower degree than the denominator. Long division makes this magic happen and then logs come into play in actually evaluating the integral. At least one of my students did not quickly recall long division. But, rather than ask me for a refresher, Allen rewrote the problem as follows:

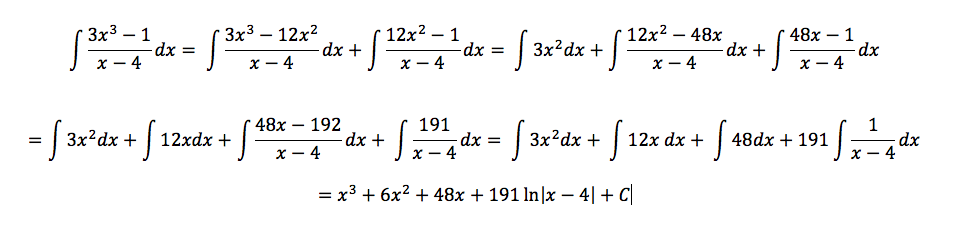
He explained his reasoning and I will paraphrase as well as I can from a conversation that happened more than two weeks ago. He recognized that the numerator had a leading term that could be expressed as a multiple of the leading term in the denominator. So he introduced a term in the numerator (and then subtracted the term he introduced) so that he had a leading piece of the integral that is a simple polynomial. The 12x^2 term now served as a multiple (again!) of the leading term in the denominator. Simply adding and then subtracting 48 x allowed him to simplify the next piece of the integral. One more iteration (subtracting and then adding 191 this time) got him to exactly where he would have been if he remembered long division.

I have to admit that I would love it if he remembered long division, I think it is a useful skill and probably saved him a little time. However, after listening to him explain his reasoning I realize that he displayed a pretty deep level of understanding here about how this expression can be rewritten in steps. The level of analysis and understanding here (I think) far exceeds simply remembering an algorithm learned in precalculus days. This has me debating how I want to approach the instruction of long division of polynomials moving forward. I’d love to hear your thoughts on this here in the comments or over on the twitters where I am @mrdardy

Bragging About My Students

Holiday break began yesterday and I find myself with time to breathe and (hopefully) get some real writing done. Before thinking about work for January, I want to take some time to pause and reflect on some of the great stuff my kids were doing before the break.

I found this problem on twitter and shared it with colleagues and classes last week:

My memory is that this image was accompanied by a simple ‘What do you notice?’

It took me a minute or two to notice what was happening. I showed it to a colleague who started chuckling instantly. He has a faster mind than mine!

So I showed it to my classes, they all eventually noticed that the digits 1 through 9 were all used in this equation. My challenge to them was to write an equation using the digits 0 through 9, once each, that was also true. I urged them to not simply add a zero to one side of that equation above.

The kids dove into this challenge and came up with some great solutions. I have a photo I took on my iPad with some of their solutions superimposed on the image of the original problem.

The top right equation is missing the 9 on the right side of the equal sign

Fun, right? Even better is the fact that some kids were still working a couple of hours later coming up with ever creative solutions. My favorites were both cooked up by a student who had a sub in his Health class (me!) and he was tinkering with this problem at that time.

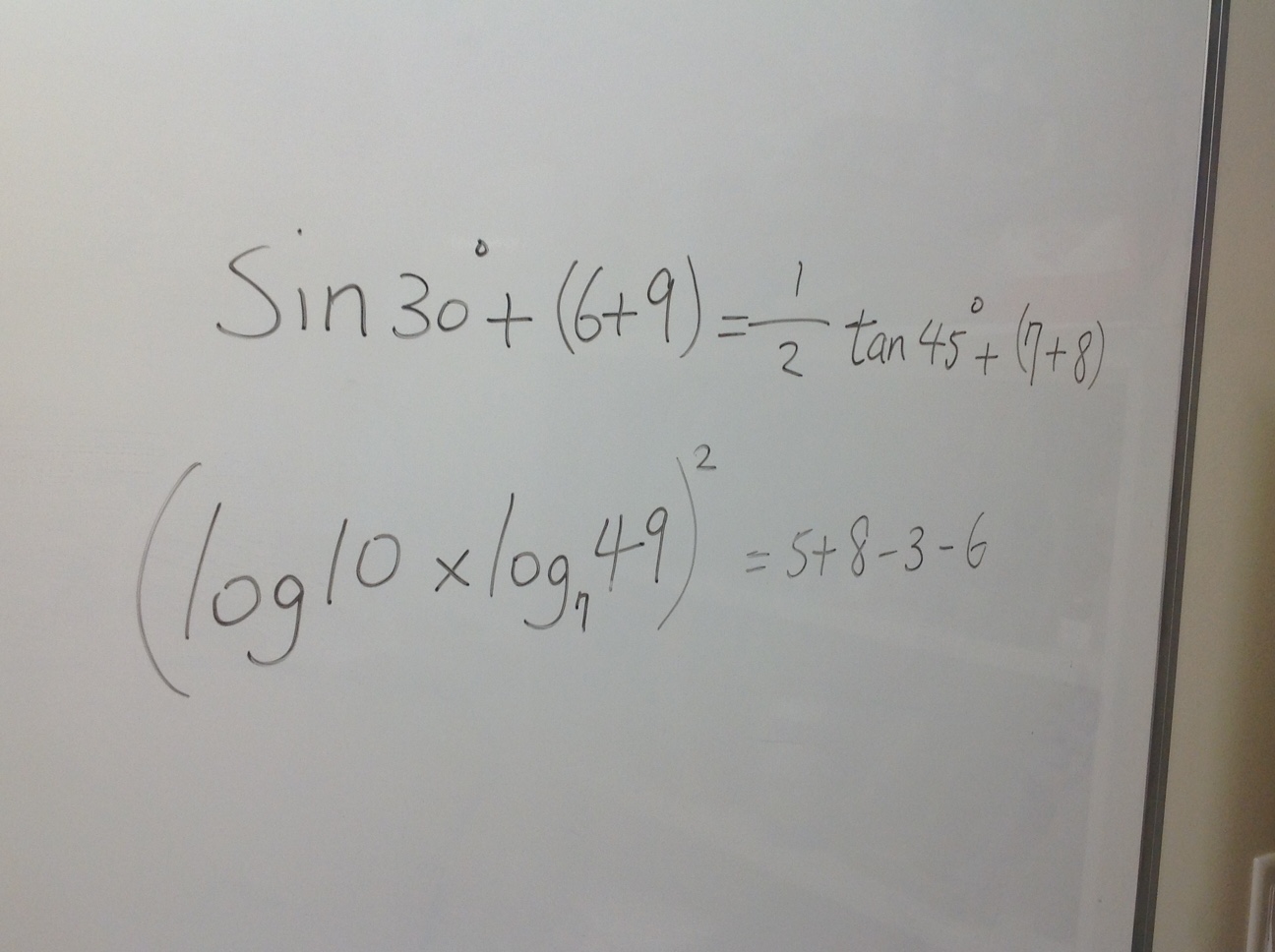
This one won my heart, I must admit.

I hope to do some more writing in the next two weeks. Some will be public, some will be piles of problem sets for my kiddos.

Empowered Problem Solving / Empowered Teachers

Not too long ago according to my calendar, but a long time ago now according to how the pace of school life moves, I finished an online workshop run by Robert Kaplinsky. The workshop, in six modules, was called Empowered Problem Solving. The modules were released on a weekly basis and were centered on videos of a workshop that Robert ran. These videos were accompanied by some outside reading in the form of blogposts and some PDFs. There were question prompts to encourage lively conversations on a message board, and there was quick support through emails from Robert and others working with him in the one or two cases early in the course when questions popped up about navigating the interface that they had set up. I did not recognize the names of folks on the message board there but I came to develop a sense of kinship through our conversations over the course of almost two months. Several themes emerged, of course, and it was interesting to go back through message boards from earlier lessons to see how my thinking was moving/growing and how the conversations deepened over that time. Looking back now, a few weeks after the course ‘ended’ [we still have access online for at least another month to revisit ideas and to help deepen our understanding/comfort with the ideas of the course] at a folder I created with documents that Robert organized for us, I realize that it will probably be out extended Christmas break when I can really digest and inject some of the habits of mind that are encouraged in the course. It made me think of my journey in grappling with/enacting/understanding the principles of inquiry and open-ended problem based lessons in the math classroom. I was forutunate to have had a Master’s Degree class in 1987 (before my teaching career began) called Mathematical Problem Solving. My grad school advisor, Prof Mary Grace Kantowski earned her Ph.D. in 1974 and her dissertation was Processes Involved in Mathematical Problem Solving, so I got a dose of this working with her and taking her class. I entered the high school classroom in the fall of 1987 and I have been honing, adapting, striving, to really figure out how to incorporate something more meaningful than practice exercises with my students. I was further energized by my first visit to the Anja Greer Conference at Phillips Exeter (I know it was between 2001 and 2005 but I cannot remember for sure what year it was) when I met Carmel Schettino and learned from her about problem solving in the math classroom and I am certain that this was my first exposure to the Exeter problem sets . The conference was mind-blowing and I was fortunate enough to attend one other time since then. Carmel’s work and advice energized me further and I started writing my own modest problem sets. Later, I wrote my own Geometry text that our school used for five years and in the process of that, I wrote HW for the course in the form of smaller problem sets. I have been fortunate enough to attend a summer think tank styled workshop that Carmel ran. I went with three colleagues to a workshop run by some folks from Packer Collegiate Institute in Brooklyn last year. I visited the Peddie School in New Jersey with three colleagues and we saw what they had done with their curriculum. Our school was visited by a member of the math department from Saint Andrew’s School in Delaware and he shared what they have done with their curriculum. All of these experiences led me to want to enroll in Robert’s online classroom and it was well worth my time and energy and the school’s investment of professional development funding. Conversations are happening in our school about the direction we want to go for our students and the visits and workshops last year helped prompt these conversations. The ideas and resources from Robert Kaplinsky’s workshop will be immensely helpful in moving this conversations forward.

All of this is a long winded way of me saying thank you to Robert, to Carmel, to the folks at Peddie who welcomed us, to Eric Finch from St. Andrew’s in Delaware, to my advisor Prof Kantowski. All of these voices throughout my career seem to be pointing the way to a more meaningful way of teaching and learning mathematics. Robert will be running his workshop again in February and March and I encourage you to take part. Whether you are just beginning to grapple with the ideas of running your classroom as a place of open inquiry and driven by problems (rather than exercises – a distinction that Prof Kantowski often discussed) or if you have been working with these ideas for years and are looking to be re-energized or more organized, this will be a great experience for you.

Thanksgiving

November is a rough month at my school with days shortening, exams looming, and temperatures dropping. I have been meaning, for some time now, to write about a fantastic experience I recently concluded with a workshop run by Robert Kaplinsky called Empowered Problem Solving. On Thanksgiving with family here, this is not the right day to write in depth, but it is the perfect day to send out a quick note of Thanks. Not only to Robert whose workshop energized me and made me think about my classroom in new ways, but to my entire community of virtual friends and colleagues. The lives of my students have been so enriched by the interactions I have had for years through blogs, through twitter, through Global Math Department chats, through workshops online, through TwitterMath Camp experiences, through EdCamps that I learned about from my online team, from classroom activities shared freely by thoughtful educators around the world, …

I will write something meaningful and targeted about my workshop experience but today I want to make a more general and wide open thanks to all those out there who have made me a better and more thoughtful teacher in the past ten plus years of blogging and tweeting.