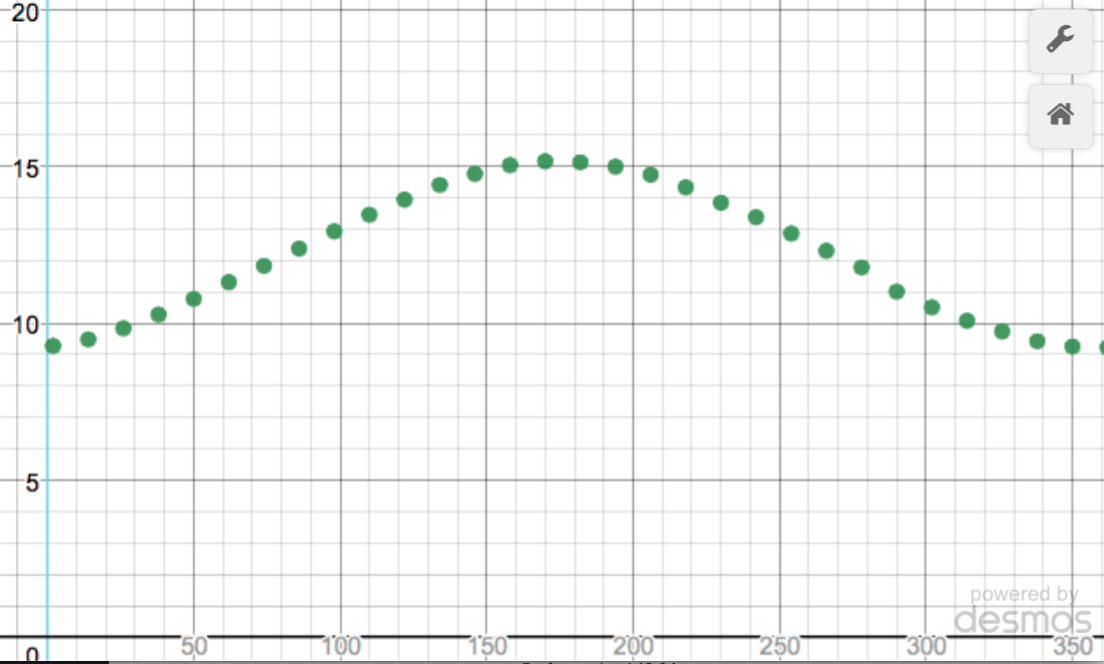
I am not 100% sold on how I have divided the data, but I want to think out loud about how my opening day activity for Precalculus Honors is developing.
Last year, I took the advice of a number of online colleagues and divided my classroom into table sets of 3 for student groups. I anticipate that I will have five of these student groups in Precalculus Honors (PCH from now on in the post) so I set up five subdivisions of the data that I previously shared (in this post) and I will start off with the cleanest of the data sets. At the bottom of the post I will attach GeoGebra file links as well as the current status of my handout. I will present screen shots of the data subsets and discuss my hopes and dreams for how this activity will unfold.
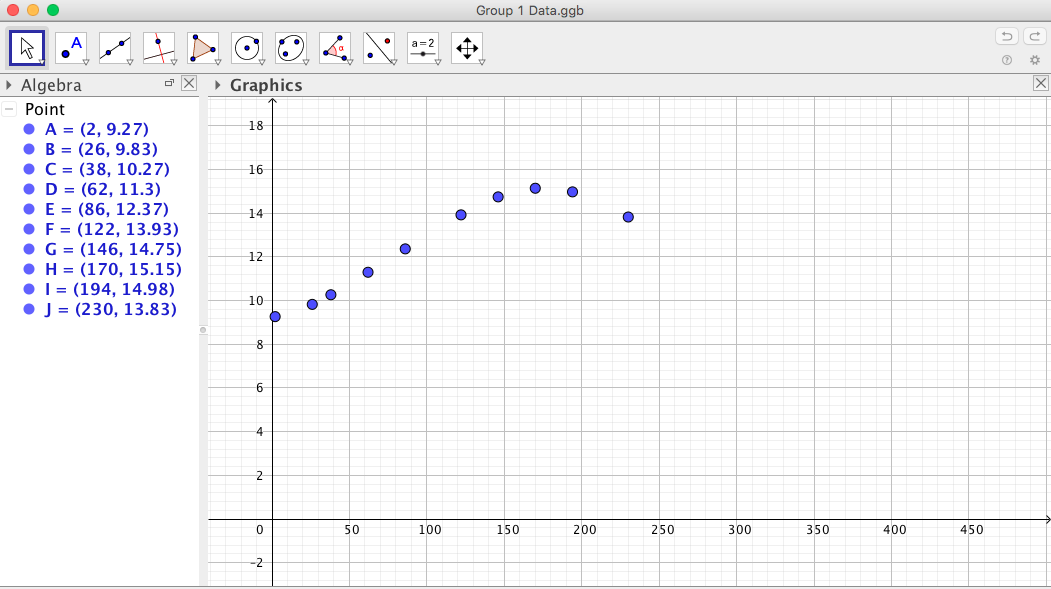
Group #1 will have an image similar to this when they graph their data. I will share this exact one with the whole class. I set up five GeoGebra files with the same screen dimensions (at least I think the dimensions are exactly the same, hope so!) My hope is that this will feel largely quadratic to them. I do not think that they have previous experience with regression equations on their TI or with any online tool. I am suggesting that they use Desmos in class. I will present GeoGebra with the goals of exposing them right away to two of my favorite tools. We’ll discuss which one might feel better for different situations. In the past few years I have had a number of students adopt both programs as a natural part of their problem solving. On more than one occasion a student has written a note on a problem set along the lines of ‘Desmos agrees with my conclusion!’ A natural direction for them to go is to use point H as a vertex and develop a quadratic that fits this reasonably well. Hopefully, we can incorporate a discussion throughout the year of the messiness of real world data compared to mathematical models. I love quoting George Box here – ‘All models are wrong, but some are useful’
 Group two will generate something similar to this image. Again, I am anticipating a quadratic guess. This time point E should be seen as the vertex. Playing a bit with my TI and anchoring my guess at point E yields the following promising picture.
Group two will generate something similar to this image. Again, I am anticipating a quadratic guess. This time point E should be seen as the vertex. Playing a bit with my TI and anchoring my guess at point E yields the following promising picture. 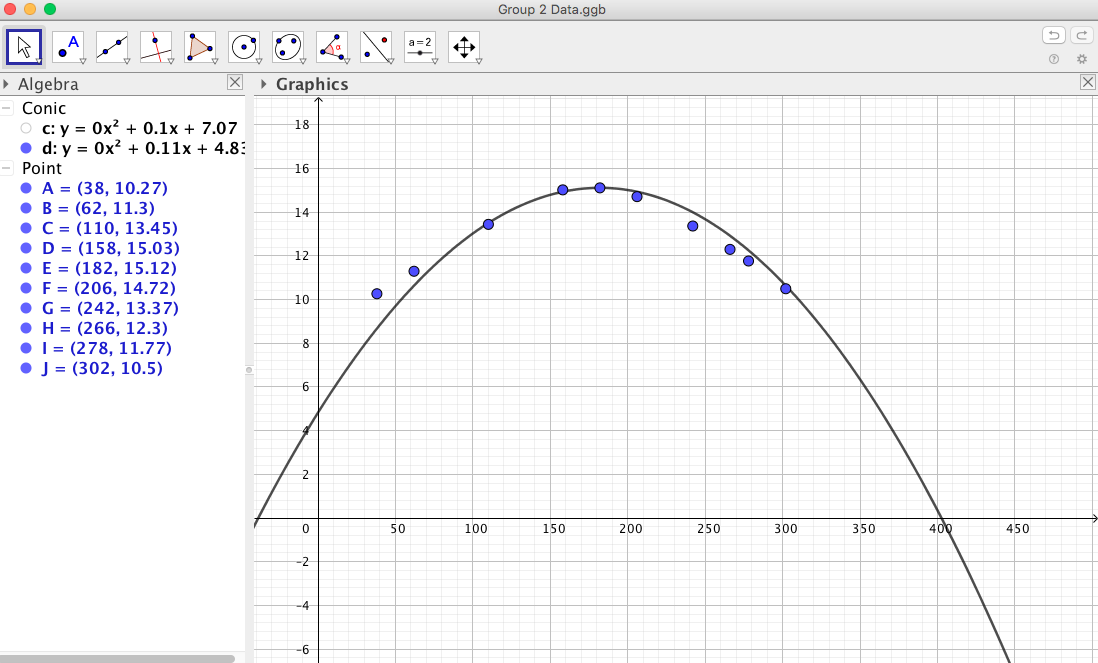
Now, we are getting somewhere!
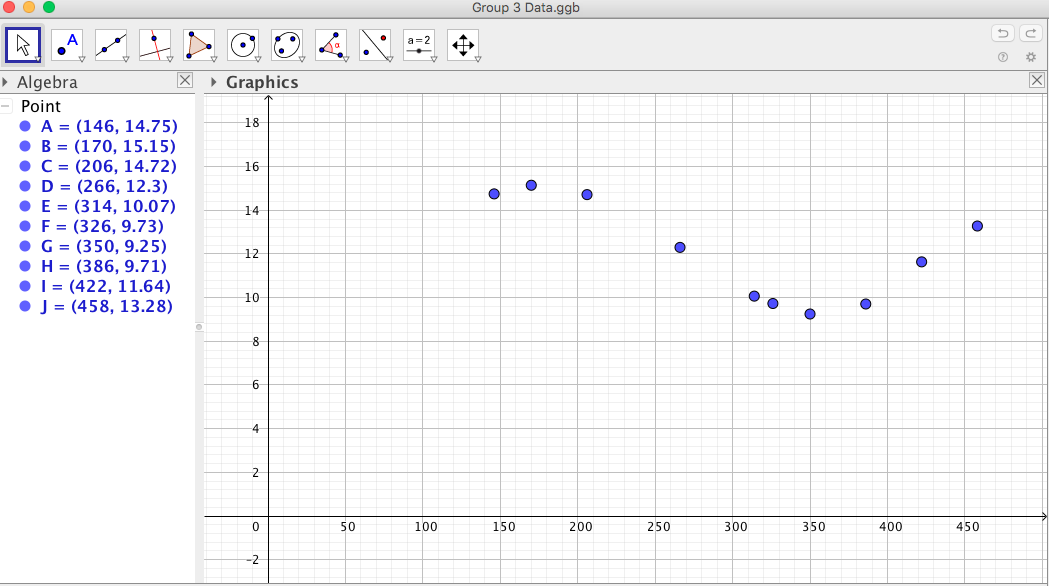
Group three sees this data and has some decisions to make. This is where I am really questioning myself. I worry that there is too much here for a first day activity. I think I may tweak this data set so that it is also more deceptively quadratic in nature.
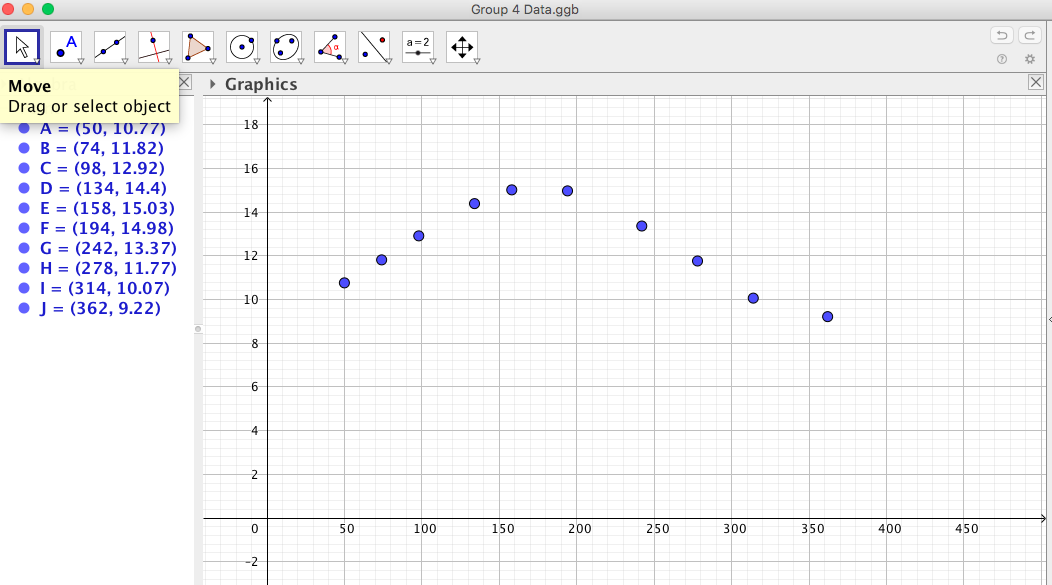
Group 4 picture above.
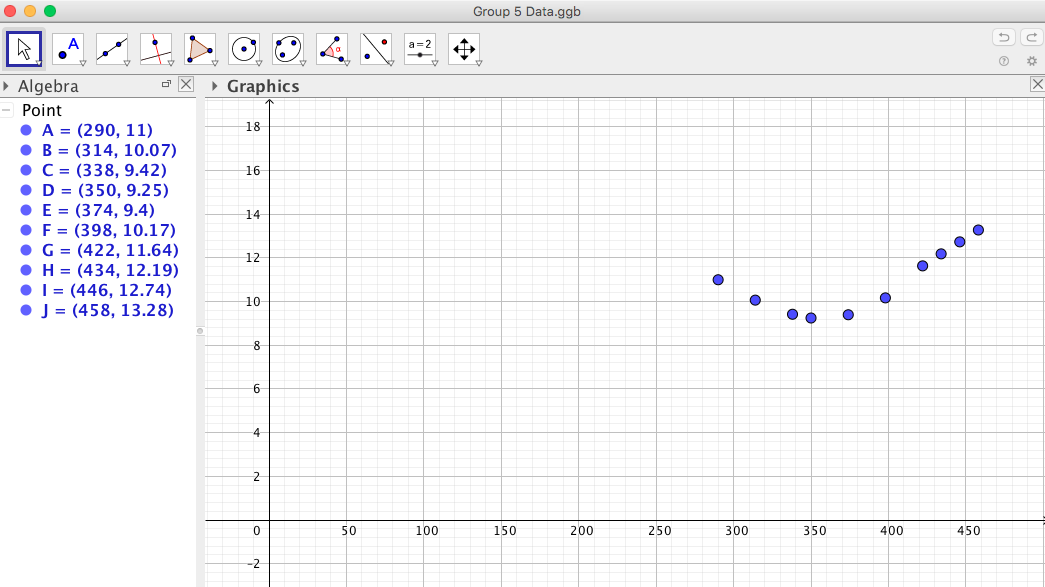
Group five data picture.
And, finally, the whole set together here –
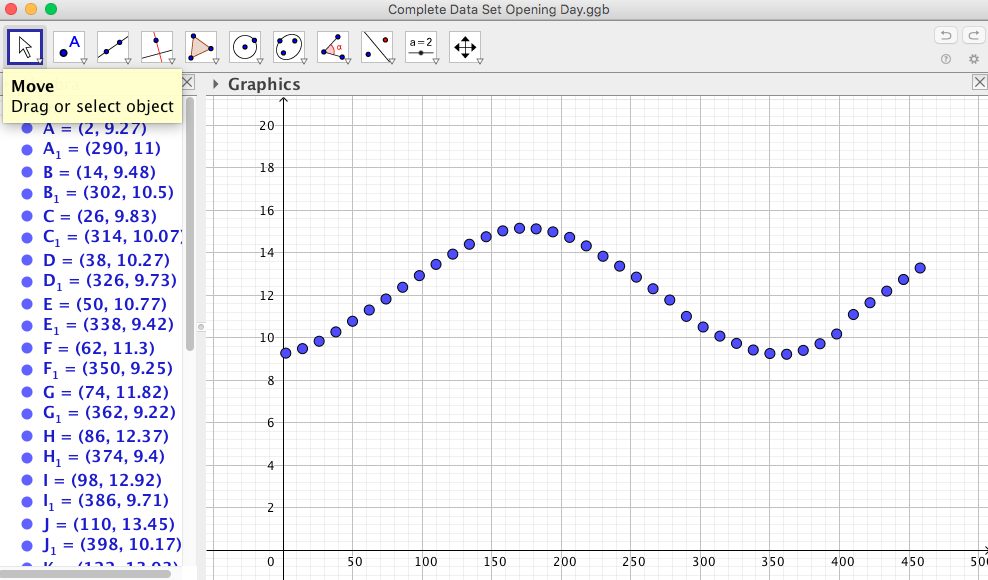
As I write this and think about my goals for that first day, I am sure that I want to modify the data that group 3 gets. I don’t want this play to become frustrating on day one. Let’s save frustration for later on!
I am hoping to plant seeds for a number of interesting conversations to have over the course of the year. I want the students to think about decisions based on small sets of data versus larger sets. I want them to think about periodicity and where/when/why to expect that behavior. I want to present them with an intentionally open question on our first day together to set a tone for open questions together throughout the year. I want them to remember something fundamental about quadratics and I expect to present two forms (standard and vertex) on the board after a little nudging from the groups.
I worry, as I often do, that I am being too ambitious. We have about 30 minutes together on day one due to a whole school convocation that day. I am really debating whether to make this the activity and conversation for our first full class day together. In that case, I would pull out an old favorite problem to have as the conversation seed for day one and mix in a bit of the boring old syllabus, etc on day one. Any advice there?
Here is a link to a dropbox folder with the GeoGebra files as they currently are arranged as well as my Word handout.
As always, advice/comments/questions are welcome here or over on twitter where I am @mrdardy