MTBos – A Portrait of a Math Teacher as an Aging Man

Thinking About Trig

In the fall I will be teaching a section of Precalculus Honors at my school. I have not taught that course in about six years so I have not spent a bunch of time thinking about teaching trig functions for awhile. Our Precalc Honors class starts off with a study of Trig and I am thinking about an opening day activity that might plant a number of seeds that we will need to germinate over time. I want to play with some data that is periodic in nature and try to generate some hopefully interesting questions. Thinking along the lines of Dan Meyer’s challenge of finding an aspirin. I tweeted a bit about this and have been engaged in a terrific conversation with Bonnie Basu (@GotMathHelp) about a similar activity that I used as a demo lesson for a job interview years ago. The context is different since those students had already been a bit immersed in their study of trig but they had not been graphing yet. My goals with that group were to uncover the periodic nature of the hours of daylight, to talk about why other functions that seemed appropriate to the picture (especially quadratics) were not appropriate, to build up some sense of important vocabulary for trig graph analysis, and to simply plant some important ideas that would be explored in greater depth after I was gone. Looking back on the activity I see all sorts of tweaks I want to make and Bonnie has been super helpful in asking questions/making suggestions. Anyone who wants to take the time to share ideas or questions would certainly help make the beginning of my precalculus class this fall more meaningful and successful. I thank you in advance for any tips.

After another round of conversation on twitter that included tips from a student who just graduated from our school, I have been playing with three different data sets. I want to play with average daily temperature, high tide level, and with daylight hours. The data for the first two came from wunderground and the third data set came from dateandtime. Below are pictures of the data and Desmos links to the tables.

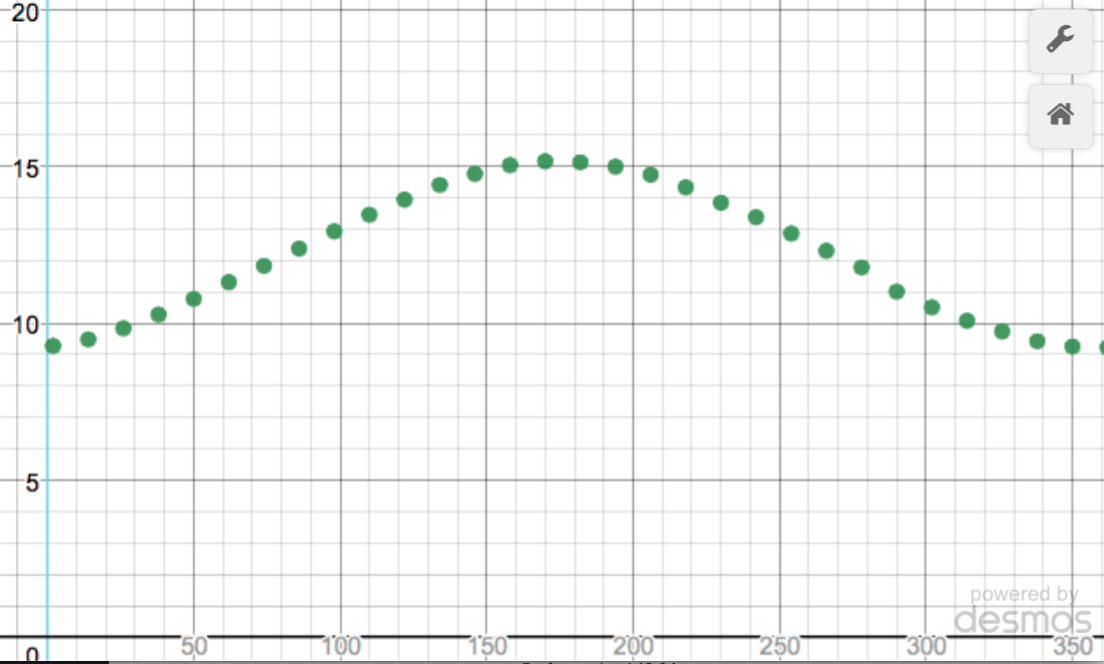
This picture (above) shows the average daily temperature in my town during 2017 at 10 day increments. You can see the Desmos table here. There are some things I like about this picture. I like the fact that the general shape can be inferred. We can talk about why it fluctuates on a number of different levels. However, I don’t think that this is a great data set for beginning to develop an idea of periodic functions. It feels too noisy to me.

Here is the picture for high tides.

This table was built on data at 6 day intervals from the beginning of the year through June/July. You can see the Desmos link here. I would definitely ask the students to play with window sizing and I think that some powerful ideas about amplitude and vertical shift can quickly come out of such a conversation. I picked 6 day increments thinking that I would be slightly off phase with what I thought would be a period related to the full moon. A quick survey on google just talks about a 12 hour plus period so I may have been making this up in my mind. This picture feels more friendly with just a little noise involved. I might use this one early – maybe even on day one. The next one is much cleaner looking. Here is the data on length of daylight hours during 2017.

This is where my mind was when I created the demo lesson. However, this data is for our hometown here. You can see the Desmos link here. My thoughts about this data set go down two different paths. One thought is that this is clean and clear and easily explainable. One tweak I might make based on my conversation with Bonnie is that I might extend past one full year (say about 400 days or so) to make the periodicity visible not only intuitively meaningful. My second thought is that this might be too clean that it might lead my students into expecting such clean, clear periodicity in a messy world. I am probably overthinking this on the second train of thought.

I expect to have five table groups of students (groups of three in my classroom after long debating it, I accepted the wisdom shared by a number of MTBoS folks – especially Alex Overwijk (@AlexOverwijk) and my classes were better this year because of that change!) and I am thinking that each table group should have different data. I am playing with the idea of mixing up sunlight or tidal subsets of data versus simply subdividing one larger set. For example, if I go with the cleaner daylight data I can extend it to about 450 days or so and give different table groups subsets of about 35 data points each. I feel that they would benefit from seeing how the data ‘fits together’ and that individual table group decisions about amplitude, vertical shift, and period all match each other pretty well.

I would love some feedback/suggestions/questions and I thank Bonnie again for her valuable thoughts. You can drop comments here or over on twitter where I am @mrdardy

Taxicab Geometry – A Brief Exploration

We are officially on spring break here at my school and we end the term with a week of what are called test priority days. The idea from the school’s end is that we want to protect students from having says with three (or more) major assessments as the winter term comes to a close. With a two – week break most teachers try to put a little bow on their material before taking off so as not to simply start off again on March 14 repeating a week’s worth of material. However, this leads to some awkward scheduling. My last test priority day was Monday and I met classes on Tuesday, Wednesday, and Thursday. I sent out a call for ideas on twitter (like you do, right?) and I received a handful of great suggestions. From a conversation with Henri Picciotto (@hpicciotto) and Becca Phillips (@RPhillipsMath) I decided to spend a few days with Geometry AND with Discrete Math on an intro to taxicab geometry. Henri shared a great link to one of his pages (I encourage you to download that file from Henri – the relevant ones for this discussion are labs 8.4 – 9.1) and I modified some of those ideas and created two handouts of my own (here is #1 and here is #2)

I want to take a moment here to reflect on how our two and a half days with this unit went. We worked Tuesday and Wednesday in each class and wrapped up our conversations before tackling the cool problem I wrote about here to finish our time together on Thursday. First, I want to comment on my documents and how I intend to tweak them before using them again. Then I will comment on the class action these days.

Handout #1 – First change I would make is that point B would be the point (5,4) instead of (4,5). I do not know if any student caught this, but when I imposed the map of Gainesville, FL on the situation I described, Anne is not at the point (4,5). This tweak would solely be for my comfort. I do know that students in all three periods had trouble deciding whether street location should be an x coordinate or a y coordinate. It should have been an easy decision, I think. I like the introduction of the Manhattan map as a way to discuss what a city block might mean, but I did too much talking this first day. I need to introduce the idea then get out of the way and let the students ask these questions. I also should change some of the coordinates I suggested. My students really wanted these points on the section of the grid I provided. I should probably adjust for that. Finally, I have to admit that I am pleased with the questions I asked here. I think that there is a pretty nice balance of practice, of comparing taxicab and Cartesian distances, and of asking some nice guiding questions. By the end of the day Tuesday I felt that my students were in a pretty good place.

Handout #2 – I like that I start off with the same image and the same text to reframe the conversation. I think I will take away the text here that defines a circle and make sure that this definition arises from conversation – either whole class or in small groups. I love the sense of discovery that emerges as the students begin to realize what a taxicab circle will look like. I had GeoGebra fired up on the projector and started taking ordered pair suggestions so we saw the shape emerge together. I am happy again with my questions here even though I am unsure of whether there is actually a clear formula for the number of lattice points inside the border of a Cartesian circle. We did stumble upon a formula for the lattice points inside a taxicab circle and it was pretty darned exciting to see this unfold. Since this was the last night of class work I had very little evidence that any of my students had entertained this question on their own. We had a nice enough conversation about it in class, but it would have clearly been more energetic if there had been some reflection on their own by any of my scholars.

My Geometry students seemed more engaged and interested in how the ideas unfolded in this exploration. Perhaps this is due to its clearer relationship to our ‘normal’ material for the course. My Discrete kiddos were willing to have these discussions, but they were clearly less excited/annoyed/engaged/frustrated/surprised by the discovery of the fact that circles are now squares. I felt pretty committed to the idea that we should agree on whether we wanted to limit ourselves to only considering lattice points when deciding about the nature of the taxicab circle. I had been rooting for a loosening of the idea of points here so that we would have a continuous boundary in the taxicab world as we do in our Cartesian world. Since I had so clearly framed the conversation the day before in terms of city streets and avenues almost all of my students wanted to stay with that restriction and they voted clearly to restrict to lattice points. There have been a few other places where the Geometry students were asked to agree on definitions. We agreed that a trapezoid should have only one pair of parallel sides and we agreed that kites should not have four congruent sides, they should have two pairs of congruent sides that were not congruent to the other pair. There is a clear pattern of wanting to agree to more restrictive definitions here. I have discussed this with one of my Geometry teammates and he seems a bit bothered by my willingness to allow these restrictive definitions. I understand his point about definitions later on in math, but I feel pretty committed to letting the students come to these agreements together at this level. I hope I am not undermining their future as mathematicians here. I like the placement of this material in a short, unconnected time span on our calendar. We could have this conversation at a number of times in the year and I want to keep this in my back pocket to uncover when time allows/demands a unit such as this one. I think that the fact that the students knew that this would not be part of an immediate assessment allowed them to relax a bit and just play with some of these ideas. I also think that this fed into the near complete lack of work done on finishing the questions I presented after class discussion time. I think I am willing to accept this limitation as long as the benefit of relaxation comes along with it.

I want to thank Henri and Becca for helping push me into this and I want to thank my teammate Mary who was willing to dive in and try this unit as well. My other two teammates tried some different ideas and I want to pick their brains to see how life went in their classes for these three odd days. I also want to say that I am fairly happy to have a bit of a break now and I hope to return to school on March 14 with at least a couple of weeks planned out carefully for both the Geometry and Discrete Math classes.

MTBoS New Year’s Resolution

Every year we all experience this, right? We have big goals for the new year, we make promises and many of them fall by the wayside. I am going to be modest about my new year’s aspirations (at least in public!) and I am making a vow to myself to try something new for my Geometry kiddos this new calendar year. Awhile ago I got into a terrific twitter exchange with Henri Picciotto (@hpicciotto) and some other folks ( I wish I could remember everyone involved, I am pretty sure that Julie Reulbach (@jreuhlbach) was part of this) about HW. I mentioned that I will sometimes include problems in HW that touch on ideas we have not talked about yet in class. This brought up a conversation about leading vs lagging homework ideas and Henri is particularly articulate about these ideas over at his blog space (http://www.mathedpage.org) We tweeted about the idea of letting ideas percolate either before instruction or after instruction. I spoke about my feeling that it is important to have students struggle with an idea a bit to (maybe) help them appreciate a new idea/definition/formula when it does arrive. This, I think, is sort of like Dan Meyer’s series of if ______ is the headache, then ______ is the aspirin essays. One of the results of this terrific, spontaneous twitter chat was that I walked away with a commitment to instituting lagging HW assignments in my class. I wrote out a careful pacing calendar with the optimistic idea that I have a solid sense of how long these conversations will take and a hope that mother nature will not interfere by dumping snow on us at some point. Obviously, this calendar is not set in stone. What IS set in stone is my commitment to the pacing of the HW assignments. What I did was write out a pacing calendar that delays by three or more days any HW that directly relies on reading/instruction of a new section of my Geometry text. I do still feel a bit of a commitment to daily practice out of class – I know, this is another conversation completely – so I wrote a series of review HW assignments that reach back to old ideas/skills but I tried to do so in a way that is thoughtful and leads to preparedness for the new ideas we are discussing. I also wrote a series of entrance slips that I will start class with the day after we first discuss a section together. These will be collected and marked, but not graded. I am hoping that these will provide both me and my students a way of monitoring their developing understanding of new ideas. After three or more days they will final have a HW focused on a certain section of our text. Meanwhile, in class we will still be following a pace similar to what we followed last year in our first run through my Geometry text so I have some sense that this is a reasonable pace for our students. However, instead of going home and immediately practicing some new set of skills, they will be looking back either weeks (at the beginning of the chapter) or days (after a few days in) to ideas that have, hopefully, been percolating a bit in their brains. They will have had time to think about these ideas, they will have had an entrance slip check in on their facility with the ideas at hand, and they will have had further class time combinations of lecture and discussion to play with these ideas and build them up together. A few days afterward they will go home with focused practice and their assessments will also be lagging a bit to line up with the HW practice. I anticipate that there will be some concern expressed by my Geometry team and by my students because, you know, change is a bit of a challenge. This is especially true once you have established a rhythm and pace that you are comfortable with together. One of my three colleagues has expressed a desire to try this as well but the other two have not commented yet and I have no expectations that anyone else needs to follow me on this path. As department chair I kind of want to test the waters on this so that I can report back on the inevitable speed bumps as well as the successes that we encounter. If this works the way I think that it will, it will radically alter how I think about pacing and how my students think about HW. My biggest wish for this endeavor is that this practice will enhance retention and help my students think more about connections in the ideas we work with in class. I feel more confident about taking this leap in our Geometry course first for a couple of reasons. I feel more intimately familiar with the contours of this course since we are using a text that I wrote a couple of summers ago. I also feel that the Geometry course lies a bit outside of the vertical tower that much of our math curriculum builds. If we slow down a bit and there are not topics near the end of the course that we reach, it feels that there are fewer consequences in terms of what future courses and teachers will expect of these students. Also, these students are younger than those in my other two courses (a Discrete Math elective and AP Calculus BC) and I am optimistic that these younger students might be more flexible with the idea of changing their habits.

If you have not seen my Geometry book yet and want to take a look, you can download it from my Dropbox at this link. If you want to look at my Chapter Six pacing calendar, entrance slips, and HW assignments, you can find them all in this Dropbox folder

I am hoping that January will be a productive month for this blog space as I reflect and report on how this experiment unfolds.

Another Lovely Learning Day

This morning I had the pleasure of presenting a session at the summer conference for the Pennsylvania Council of Teachers of Mathematics. I know a few people in the organization and in a twitter conversation with Bob Lochel (@bobloch) I was encouraged to submit a speaker proposal. I wrote up a proposal for a session called Escaping the Tyranny of the Textbook. As my loyal readers know I spent the summer of 2014 writing a Geometry text for use at our school (you can find the 2nd Edition here) and I wanted to talk about the relative ease (compared to 10 or even 5 years ago) of putting together your own curriculum. I was not there to advise that people write their own books, just to point out the wealth of resources available on the web and through twitter. I was fortunate enough to have my proposal accepted and I was originally slotted to lead a small roundtable conversation for about 30 minutes. Well, about a week ago Bob reached out and asked if I was willing/able to move to a 50 minute time slot. I agreed but figured that this required a bit more advance planning on my part. I sent out a call for help and received numerous helpful tweets and I put together this Power Point slide show. I had a group of about twenty people in the room and we had a lovely conversation. People asked some great questions, they seemed to appreciate the resources I organized with the help of the MTBoS tribe, and I have already had folks reach out to me via email. The common theme that emerged was a desire on the part of the participants to have support in navigating the world of resources available. A few people told tales of schools giving them technology tools but no PD support to help them use those tools. It is clear from my experiences, and from the voices in this room, that this is a long process. Finding trustworthy connections both in your building and out in the wide world, takes time, energy, and support. I positioned myself as a cheerleader for MTBoS and for collaboration – both virtual and face to face. I am optimistic that I will strike up a correspondence with some of the folks in the room this morning and that some of them will take the jump this year to reaching out of their classroom and tapping in to the world of resources available to them.

TMC 15 Reflections, Part Two

So, in my last post I gathered some of my thoughts about the days at TMC up through the post-lunch keynotes. I did not touch on the My Favorites sessions, the afternoon sessions, or the evenings. I’ll tackle those in this post.

Each morning and afternoon we were treated to quick presentations by volunteers (well, we were all volunteers there, really) who wanted to share something brief about their classroom practice, about some project they were working on, or to share some other insights. These were all fun nuggets and I don’t want to ignore any of the treats that were shared, but I do want to single one of them out. Glenn Waddell talked about his commitment to high fives with his students. When I was a young teacher (a LONG time ago) I was encouraged to stand outside my door between classes. I try to remember this and use this as an opportunity to greet my students and other students passing by in the hallways. However, I do not have the kind of commitment to this and energy that Glenn shared. He greeted each student with a high five and discussed how he broke down the resistance of a particular student who finally joined in the fun. He talked about how on days when he had to miss class, the students would ask for two high fives the next day. I had the pleasure of picking Glenn’s brain about all of this one evening and he told me that would tell his students that he was congratulating them because they were about to do something awesome. He also said that he greeted each class with a rousing ‘Good Morning!’ no matter what time of day he met them. His enthusiasm was infectious and I could not resist giving him a high five every time I saw him the rest of the week.

I went to two afternoon sessions each day and I want to highlight two particularly fantastic ones. Dylan Kane (@math8_teacher) conducted a session called Arithmetic to Algebra: Key Building Blocks in Abstract Thinking. Dylan shared a series of fantastic questions that he had developed to extend student thinking and give him insights into their thought process. Dylan is bubbling with energy and enthusiasm and he asks great questions. He has been on fire on his blog lately – if you have not read his stuff you should change that and visit him regularly. A great conversation ensued in the room and I am looking forward to using some of his questions as class warm ups this year. Meg Craig (@mathymeg07) conducted a session called Function Transformations Without Tears. I have raved about Meg and her blog recently where she has been on a tear recently sharing tons of great files. She conducted a thought provoking session on how to approach function transformations together. What was as impressive as the thought that went into her presentation was the energy of the conversations in the room. A number of us were sharing ideas about what language to use and how to incorporate this into our classroom. Meg gave us room to debate/discuss even though it may have taken time away from what she was thinking of doing in her session. Both Dylan and Meg stepped back and let us, their ‘students’, take the conversation where we needed/wanted it to go. Nice modeling of positive teaching behavior on their part!

This just leaves dinner time and evening fun to recount. On Thursday I had the pleasure of meeting my brother for dinner. It has been well more than a year since we had seen each other. He has lived in LA for nearly twenty years and just recently bought a house outside the city. We met in a nearby town that was roughly equidistant from his home and my hotel. We were able to spend nearly four hours catching up before I started winding down and headed back ‘home’. When I arrived at the hotel a little after 10 PM I walked into the hotel courtyard to find about 40 or so conference members hanging out and catching up with each other. I was greeted warmly by three of four folks as soon as I walked through the doors and my energy was lifted. I spent some time chatting – especially with Brian Miller (@TheMillerMath) who I met last summer. Brian became my car buddy for a number of events over the time there and I tried to help him with an unfortunate battery problem his car developed. I was struck once again by how meaningful friendships can feel even though we had seen each other in person for only three days in Oklahoma. Meaningful conversations with Alex Overwijk (@AlexOverwijk), Jasmine Walker (@JazMath), and others over the time there made the hotel courtyard a warm and wonderful place in the evenings. One evening featured a barbecue at a nearby park that was funded by the wonderful folks over at Mathalicious and prepared by local hosts. It was a beautiful night to be outside, great food, good conversations, and wonderful views of the mountains nearby. Having spent most of my adult life in Florida, I am taken by mountains.

On my final night there I ended up having dinner and a cupcake run with a group of 8 terrific women (lucky me) before retiring to the hotel courtyard again. I had a very early flight back across the country but I kept myself up until almost midnight talking with fascinating folks before finally retiring for the night.

I realize I am just barely scratching the surface of the energy and spirit of the camaraderie of the folks there. I will try to capture it this way. I left for California on a Wednesday and the rest of my immediate family left the Friday before for an adventure. I was away from my wife and kids for nine days and missed them terribly. While I was at TMC15, instead of primarily dwelling on missing my family (which I still did, for sure) I felt so immersed in a different kind of family. Sure, we are not bonded by blood, but we are bonded by a love of idea, by a love of our work, and by a generosity and, dare I say it, love for each other. Although I have spent six days or less in the physical presence of this community, I feel a deep bond of trust and friendship. I have seen over and over again how a blog post about challenges and questions in my job results in thoughtful replies and sharing of resources. I have seen how a tweet sent out about a question results in quick, thoughtful replies. I have seen how the simple accident of sitting in the same place in an auditorium for three days in Oklahoma leads to a sense of camaraderie that spans the width or height of our continent. I have also seen how my wife is already making plans for me to attend TMC16 in Minnesota and how to turn it into an extended family adventure. She sees what I clearly feel. This professional community helps me grow, helps me feel supported, and tickles my brain.

Next challenge – a new school year starting in seventeen days!

Highlights of a Stressful Week

So, there have been many scattered thoughts on my mind in the past week but there are also three things that happened that are just completely awesome.

My pal John Golden (@mathhombre on twitter) steered a number of his teacher training students over to a post on my blog and twelve of them chimed in with comments. Totally cool! One of them decided to follow my blog and I took the time to respond to each of them. LOVE the idea of new teachers in training dipping their toes into this rich world of teachers blogging and sharing. I am also flattered that John thought my virtual home here was worth a visit.
I woke up Wednesday morning with a message on twitter from a teacher in Louisiana who asked if he could use my Geometry book at his school next year. I am so excited by the idea that this work might be used at another school.
In my Geometry class this week we are talking about angle and arc relationships. One of my students stayed after class one day this week and she had this to say. “You know, I was thinking, when will this be important? I mean, when will I need to find an arc length like that? Then I realized that the work we are doing to find that length is what is important. Pretty cool.” Wow.

Borrowing from the MTBoS

I’m guessing that most of you reading this are familiar with the awkward acronym for the Math Twitter Blog-o–Sphere – one of the joys of tapping into this community is that they are remarkably generous about sharing ideas and resources. Today in our Geometry classes (I teach only one of the five sections we have at our school) we used an activity written by Kate Nowak (@k8nowak on twitter). It is an activity based in GeoGebra and allows the student to explore the ratio between lengths of legs in a right triangle. You can find the document we used here . I modified (very slightly) the document that Kate originally posted here. Next time I use it I will tweak it a bit. I have only twelve students in my class and chose not to explicitly team them up. They talked with their neighbors as they are usually encouraged to. However, the directions either need to be tweaked so that team references are excluded or I need to clearly team them up. I am also debating question 7. A number of students did not make the explicit leap from using the ratio they found on page 1 and using it here. I don’t necessarily want to give away too much but I may add a little prompt that they should consider the work that they have already completed. We set up a google spreadsheet and in the next couple of days I will refer to this repeatedly to show that different students working on different triangles were arriving at the same ratio. We make a big explicit deal about scale factors between similar figures. I do not think we spent enough time pointing out that scale factors within figures will also match up for similar figures. I will definitely make this more of a point of emphasis next time through my text.

I cannot thank Kate enough for sharing this activity. My students worked well and I am convinced that they will have a more solid grasp of trig ratios moving forward. As I plan out the rest of the unit I am also going to be borrowing from Sam Shah’s latest post about trig. You can find that over here.

Man – the benefits my students are reaping from people that they will never meet – such as Kate Nowak, Jennifer Silverman, John Golden, Jed Butler, Sam Shah, Pamela Wilson, Meg Craig, and so many more – is just remarkable.

Why Do I Blog?

Once again, Dan Meyer has me thinking. This time the blame can be passed along to Michael Fenton. Michael raised a question on twitter. His question to Dan was

Could you have written a list of 5 reasons you blog 5 years ago? And a list of 5 reasons you blog now? Lists match? What’s changed?

As usual, Dan’s page is generating some great responses. You can jump to that page here.

As I write, I am on my first day of spring break. It is a quiet, beautiful morning on my mom’s back porch with everyone else sleeping. Let’s see if I can make sense of this question.

I am a relative newbie to this blogging business. My first post was in the first week of July 2013. This is my 58th post. I started reading blogs with some regularity and subscribing to them a few years ago. The first one I subscribed to for regular delivery was either Dan’s blog or Sam Shah’s. My memory is not sharp enough to recall which was first ( Edit – A little research shows that I subscribed to Sam’s blog in Oct 2010 shortly after relocating to NE PA). I was living in NJ where I moved after leaving FLA. While I was in FLA I was enrolled in a program to earn a doctorate in education. I was taking night and weekend classes while teaching full-time and raising a little boy. I even took a year off from the classroom to be a grad assistant and full-time student. I worked on a super cool research project looking at arts integration in schools and was feeling super energized by the reading I was doing, by my classmates, and by my professors. We headed off to NJ where I was going to carry out my dissertation research (where I was lucky enough to work with this guy as one of my research participants) and I worked with a really inspiring Associate Head/Director of Studies and some other good colleagues there. But, once I was back in the classroom walls, I started realizing how much I missed the level of conversation from my graduate classes. As most of you reading this know, we spend TOO much of our time in our four walls closed off to our colleagues. As many of you have probably experienced, there is not much in the way of structured time during the day where we can have meaningful conversations about what goes on in those walls. As a math teacher, I get too much semi-snarky jokes when I try to talk about my class at the lunch table or in the faculty lounge. There is something about the pace and structure of our days that seems to work against built-in reflection time. So, I found that time and space out on the internet. For a few years I read blogs and occasionally commented on them. I finally took the plunge last July and started my own. In the fall when the MTBoS blogging initiative kicked in for its second year, I was all in. I even took the plunge into twitter based on one of the challenges presented there. That must have been in October. Since then I have sent out almost 1300 tweets. All but about 50 of them have been to people I know only through my math experiences or through their blogs and tweets. The majority of them have been sent to people I’ve never met. Through these experiences, I have had the pleasure of being invited to my first EdCamp – with another on the way. I have been invited by the amazing Tina Cardone to take part in a workshop presentation at this summer’s Twitter Math Camp, I have received tweets as answers to questions from Ketih Devlin and Steven Strogatz, I have shared amazing lessons from people I’ve never met, I have pestered my colleagues with emails and document attachments that I have gathered from the web. I spend the first 45 minutes or so of each morning (after I feed the cats and start some coffee) reading my email alerts from the previous night, scanning what might have happened on twitter after I fell asleep, and checking my wordpress reader. I go to school every morning thinking about something that I might not have thought of on my own. I look forward to this quiet time in the morning before my family wakes up as a time to wake my brain up and recharge it. It’s a way to improve the lives of my students and to help ensure that I don’t feel stale and bored. I am in my 27th year in the classroom and I feel as energized about it as ever and I think that much of the credit belongs to a world of people I’ve never met.

So, I realize that most of what i have just said explains why I read blogs and prowl twitter. Why do I write my blog? A much simpler answer. I think that there are two reasons.

I want to give back – at least a little – to this rich world of ideas.
I want feedback on my ideas as they develop.

Not profound, but it feels good to chime in. Now, I’m off to breakfast.

Summertime

That’s right. It’s wintery and snowy here in NE PA and I am thinking about the summer. It’s not because I want this school year to end in a hurry. I am enjoying my classes and students too much for that. No, I’m thinking about the summer because an amazing opportunity has presented itself. Tina Cardone – she of the terrific Drawing on Math blog and the mastermind / editor of Nix The Tricks recently reached out to me and asked me to attend Twitter Math Camp ’14 and to help her in running the Precalculus offering in the mornings that week. I’m flattered beyond my ability to put it in words. Looking at the list of people who’ll be there makes me think I need to go around with a sharpie to get autographs on my lovely MTBoS shirt that Jen Silverman designed. We worked up a description of what we hope to accomplish through a lively google doc discussion. We ended up with the following

Current and future PreCalculus teachers! You are invited to join us for a workshop centered around collaboration. There will be time for looking back (topics from Algebra 2 that need reinforcing), looking forward (what will students need for Calculus) and looking side to side (topics students should study to be proficient in math as well as appreciate the fun and beauty of the subject). Be prepared to rave about your favorite topic and rant about your most dreaded one. After that’s off your chest, we’ll get down to work with enthusiastic and creative colleagues. You’ll win them over with your favorite lesson ideas and you’ll find some exciting ways to present your dreaded topics.

Not sure if your course falls under the title of PreCalculus? Tweet us and ask!

Tina @crstn85 and Jim @mrdardy

The camp meets in Jenks, OK (near Tulsa). I have felt so energized by the connections I’ve been making with many of the people I’ll finally meet there. Can’t wait and I am doing a quiet countdown in the back of my head already.